c4d.Matrix
¶
See also
Warning
The
Matrix
type does not support the function
copy.copy()
.
Instead use the
Matrix
constructor:
import c4d def main(): if not op: return mat = op.GetMg() print "Matrix:", id(mat), mat ref = mat print "Reference:", id(ref), ref copy = c4d.Matrix(mat) print "Copy:", id(copy), copy if __name__=='__main__': main()
c4d.
Matrix
¶
Matrix.__init__()
Class Constructor
Matrix.__str__()
Function
str()
Matrix.__add__()
Operator +
Matrix.__sub__()
Operator -
Matrix.__mul__()
Operator *
Matrix.__div__()
Operator /
Matrix.__invert__()
Operator ~
Matrix.__eq__()
Operator ==
Matrix.__ne__()
Operator !=
Matrix.
__init__
(
[
off=Vector(0)
[
,
v1=Vector(1
,
0
,
0)
[
,
v2=Vector(0
,
1
,
0)
[
,
v3=Vector(0
,
0
,
1)
]
]
]
]
)
¶
Matrix
.
All arguments are optional so it is possible to create a new matrix without any arguments. All components are simply set to:
mat = c4d.Matrix() # => Matrix(v1: (1, 0, 0); v2: (0, 1, 0); v3: (0, 0, 1); off: (0, 0, 0)) mat = c4d.Matrix() mat.off = c4d.Vector(100,0,0) v1 = c4d.Vector(3) v2 = c4d.Vector(12,4,9.3) v3 = c4d.Vector(9,80,3) off = c4d.Vector(v1+v2) mat = c4d.Matrix(off,v1,v2,v3)
| Parameters: |
|
|---|---|
| Return type: | |
| Returns: |
A new matrix. |
Matrix.
__str__
(
)
¶
Returns the Matrix as string. Called if
str()
is wrapped around an instance of
Matrix
. (See
__str__
):
v1 = c4d.Vector(3) v2 = c4d.Vector(12,4,9.3) v3 = c4d.Vector(9,80,3) off = c4d.Vector(v1+v2) mat = c4d.Matrix(off,v1,v2,v3) print mat # => Matrix(v1: (3, 3, 3); v2: (12, 4, 9.3); v3: (12, 4, 9.3); off: (15, 7, 12.3))
| Return type: | str |
|---|---|
| Returns: | The Vector as string. |
Matrix.
__add__
(
self
,
other
)
¶
Adds two matrices.
| Parameters: | other ( c4d.Matrix ) – The other matrix. |
|---|---|
| Return type: | c4d.Matrix |
| Returns: | The result matrix. |
Matrix.
__sub__
(
self
,
other
)
¶
Subtracts two matrices.
| Parameters: | other ( c4d.Matrix ) – The other matrix. |
|---|---|
| Return type: | c4d.Matrix |
| Returns: | The result matrix. |
Matrix.
__mul__
(
self
,
other
)
¶
Matrix
, multiplies the left hand matrix by the right hand matrix.
If the left object is of type
Matrix
and the right one of type
Vector
, multiplies the vector by the matrix including any translation in the matrix.
If the left object is of type
Vector
and the right one of type
Matrix
, multiplies the vector by the matrix including any translation in the matrix.
If the right object is a number, multiplies each vector of the matrix by it.
| Parameters: | other ( c4d.Vector or number ) – The other argument. |
|---|---|
| Return type: | c4d.Vector |
| Returns: | The result matrix or vector. |
Matrix.
__div__
(
self
,
other
)
¶
Divides each element in the matrix by a scalar.
| Parameters: | other ( float ) – The scalar. |
|---|---|
| Return type: | c4d.Matrix |
| Returns: | The result matrix. |
Matrix.
__invert__
(
)
¶
Inverts the matrix.
| Return type: | c4d.Matrix |
|---|---|
| Returns: | The inverted matrix. |
Matrix.
__eq__
(
other
)
¶
Checks if two matrices are equal.
| Parameters: | other ( c4d.Matrix ) – The other matrix. |
|---|---|
| Return type: | bool |
| Returns: | True if matrices are equal. |
Matrix.
__ne__
(
self
,
other
)
¶
Checks if two vectors are not equal.
| Parameters: | other ( c4d.Matrix ) – The other matrix. |
|---|---|
| Return type: | bool |
| Returns: | True if matrices are not equal. |
Matrix.
Normalize
(
)
¶
Normalizes the matrix.
Matrix.
GetNormalized
(
)
¶
Calculates the normalized matrix.
| Return type: | c4d.Matrix |
|---|---|
| Returns: | The normalized matrix. |
Matrix.
Mul
(
v
)
¶
Multiply the vector by the matrix, this includes any translation in the matrix.
| Parameters: | v ( c4d.Vector ) – The vector to multiply. |
|---|---|
| Return type: | c4d.Vector |
| Returns: | The result vector. |
Matrix.
MulV
(
v
)
¶
Multiply the vector by the matrix, this does not include any translation.
| Parameters: | v ( c4d.Vector ) – The vector to multiply. |
|---|---|
| Return type: | c4d.Vector |
| Returns: | The result vector. |
Matrix.
GetTensorMatrix
(
)
¶
Returns the matrix’ tensor.
| Return type: | c4d.Matrix |
|---|---|
| Returns: | The tensor matrix. |
Matrix.
Scale
(
v
)
¶
Performs uniform matrix scaling (float) or non-uniform matrix scaling (vector).
| Parameters: | v ( c4d.Vector or float ) – The scaling scalar. |
|---|
Single precision matrix mathematics - A matrix is basically an array of number arranged in a rectangular pattern. The matrices used in Cinema 4D, represented by the class Matrix, consist of three rows and four columns, i.e. they have the dimension 3x4. The first row is always “1, 0, 0, 0”, which means that there are 12 numbers that are used. These numbers are grouped into four vectors, one for the remaining numbers in each column. The four vectors are called off, v1, v2 and v3, together these four vectors can be used to represent the coordinate system for a Cinema 4D object. A coordinate system consists of three axes, one for each coordinate (X, Y and Z). The system also has a base position, from which the three axes originate. This base position is stored in off, and the three axis vectors are stored in v1, v2 and v3 respectively. Also check out Matrix Fundamentals . So now we can follow the white rabbit - knock knock - neo.
Note
Cinema 4D uses a left-handed coordinate system.
Here is a small example how to use the type Matrix:
v_1 = Vector(30) v_2 = Vector(1.5, 9.87, 2) v_3 = Vector(0) off = Vector() #Vector with components: 0 mat = Matrix(off, v_1, v_2, v_3) #create new instance of Matrix print mat.v1.x #return 30
With R12 you probably have noticed that
BaseObject
no longer has members like GetPos, but instead twice as many:
BaseObject.GetRelPos()
and
BaseObject.GetAbsPos()
.
To understand what’s going on I’ll quickly introduce you to Freeze Transformations.
For (character) animation it is important that you can define rest poses. Think e.g. of the typical CG human that has arms and legs stretched. Most objects carry all kinds of complicated position, scale and rotation information. Freeze Transformations allow you to “freeze” this information, but reset position/rotation to 0.0 and scale to 1.0. This is great as it allows you at any time to go back to the original - just reset all position and angle information to 0.0 - and your object has reached its rest state again. You could achieve the same by adding for every single object in your hierarchy a parent null object, but Freeze Transformations let you do this without the need to change the hieararchical structure. Technically however you can think of it as a “parent null object” without the actual body of a physical object.
All parts of the application that deal with keyframing only access values without the freeze transformation part. This way you can conveniently animate (the rest pose is always 0.0 for position and rotation) and avoid rotation singularities. The correct routines for animation and keyframing are labeled “Rel”.
Other parts of the application however (e.g. like a target expression) need to get the absolute local position of a target - the position that consists of frozen and regular matrix. For those purposes you need to use the routines that are labeled “Abs”.
To clarify: as long as an object has no Freeze transformation assigned (which is always the default case when you create a new object) there is no difference between the Abs or Rel. So e.g. after creating an object it doesn’t matter which routine you choose. If you however want to read the position of an object (and that object uses freeze transformations) there is a difference.
BaseObject.GetMl()
/
BaseObject.SetMl()
and
BaseObject.GetMg()
/
BaseObject.SetMg()
always include the frozen information, thus are “Abs” versions.
After so much theory a practical example. Take a look at the cube and null object in the picture below. The null object has a position of (0/200/0) and rotation of (0°/45°/0°). The cube has a position of (0/100/0). You can see to the left its global position in space.
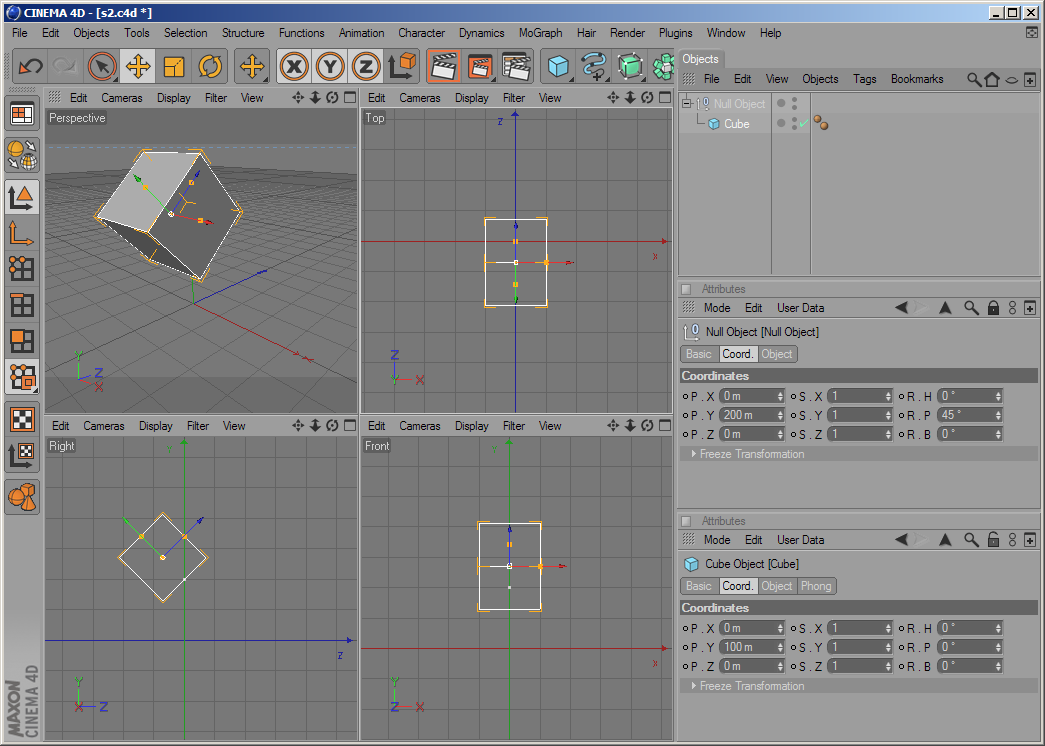
Now let’s try to do the same with one single object:
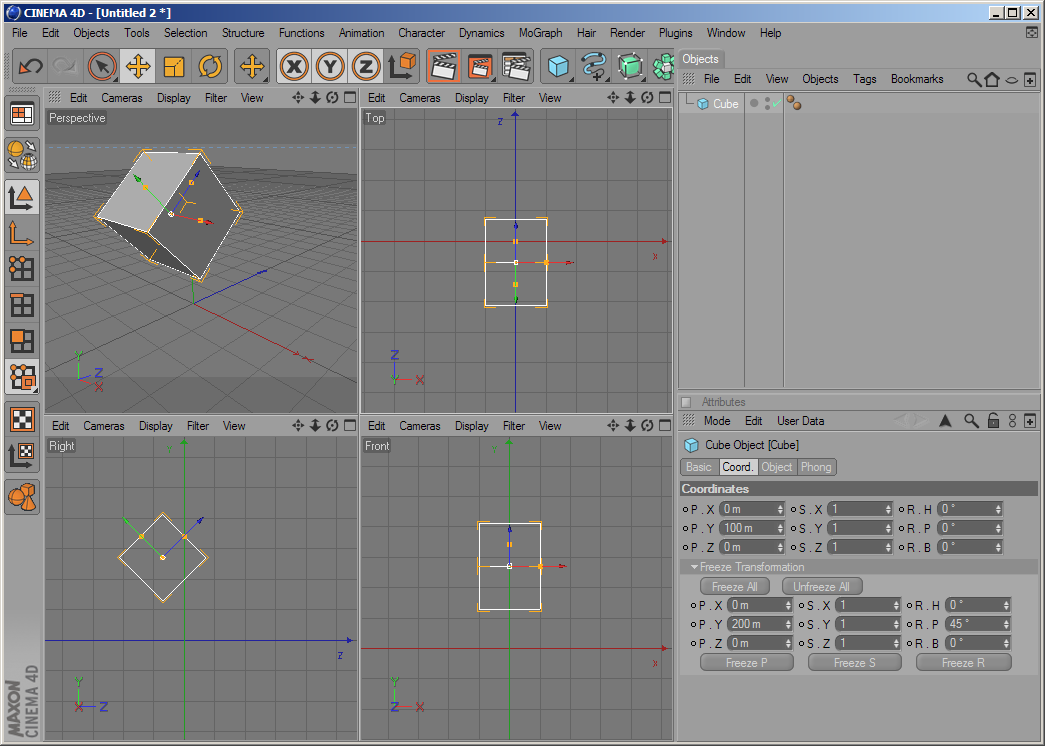
You can see that the Null object’s position / rotation have been transferred to the Cube’s frozen position and frozen rotation. The result is exactly the same as before, just without the need for an extra null object.
Now if you call cube.GetAbsPos the return value will be (0/270.711/-70.711). When you call cube.GetRelPos however you’ll get (0/100/0) as a result. The coordinate manager offers exactly those two display modes: Object (Abs) and Object (Rel).
As copying position, rotation and scale data from one object to another is much more complex than before there is a new routine
BaseObject.CopyMatrixTo()
that copies all position, rotation and scale data (including the frozen states) over to another object.
Let’s take a look at the mathematical background: In Cinema 4D the matrix multiplication order for two matrices A and B:
[C] = [A] * [B]
is defined as the rule “A after B”… so the following two lines are equivalent:
transformed_point = original_point * [C] transformed_point = (original_point * [B]) * [A]
(the original point is first transformed by matrix [B] and then later by [A])
An object’s global matrix is calculated this way:
[Global Matrix] = [Global Matrix of Parent] * [Local Matrix]
or:
[Global Matrix] = op.GetUpMg() * op.GetMl()
To calculate the local matrix of an object the formula is:
[Local Matrix] = [Frozen Translation] * [Frozen Rotation] * [Relative Translation] * [Relative Rotation] * [Frozen Scale] * [Relative Scale]
or:
[Local Matrix] = MatrixMove(op.GetFrozenPos()) * HPBToMatrix(op.GetFrozenRot()) * MatrixMove(op.GetRelPos()) * HPBToMatrix(op.GetRelRot()) * MatrixScale(op.GetFrozenScale) * MatrixScale(op.GetRelScale())
or equally:
[Local Matrix] = MatrixMove(op.GetAbsPos()) * HPBToMatrix(op.GetAbsRot()) * MatrixScale(op.GetAbsScale)
Please note that the both scales are applied at the very beginning, so the local matrix is not the same as [Frozen Matrix] * [Relative Matrix]. This is necessary in order to guarantee that your local matrix always stays a rectangular system; distorted systems would be too complicated to handle.